Vortex Math

Simple vortex diagram using modulo 9 and multiplier 2 to form the series 1, 2, 4, 8, 16 mod 9=7, 14 (or 32) mod 9 = 5, 10 ( or 64) mod 9 = 1
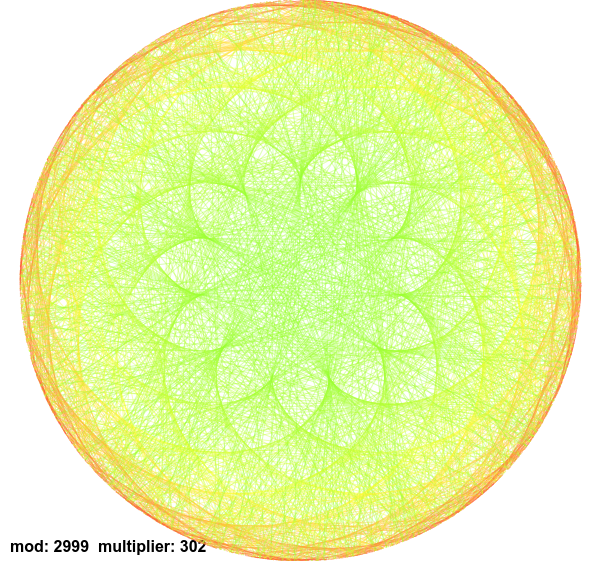
The concept of vortex math as presented by the You Tube Video seems simple enough. Just implement a function that plots a set of multiplier points evenly distributed around a circle and connections between point (n) and point (n+1) where point (n+1) = (n x multiplier) modulo modulus and point (0) = 1. Some combinations of multiplier and modulus quickly result into a repeating loop or retreat to the edge of the circle. In general there are multiplier - 1 cartioids (heart shaped figures) that form about the edges of the circle and higher values of modulus result in more definition of the cartiods. With high values of both, come interference patterns of the cartioids that form rings of increasing sizes of diamond shapes toward the center. Again it is not clear why the combination of multiplier and modulus will produce this pattern. Some do and some do not.

Vortex diagram using modulo 167 and multiplier 17 to form a 16-lobe cartioid pattern (and a 7-lobe interference pattern)
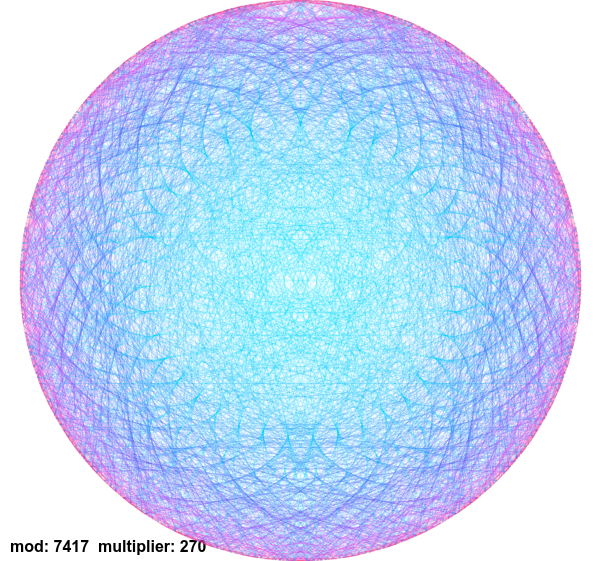
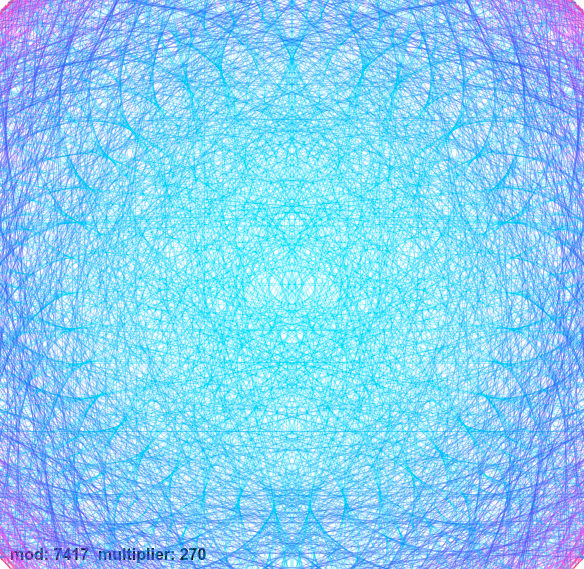
Then there is modulus = 7417 and multiplier = 270. This produces the pattern of decreasing diamond shapes that are radially stretched out. In staring at the center portion of this image, one can see faces start to appear of various sizes and temperaments. Perhaps this is something like a Rorschach test to provide some sort of insight into one’s sole. It is a mystery why a pure mathematical algorithm should be able to produce images that appear like faces of various animals (bats, bears, cats, lions, flies, mythical beasts, and just plain daemons) and various sizes. Part of this effect may be the human ability to see faces in inanimate objects like tree bark or clouds. Part of it may be because the pattern of the vortex math oscillates between clockwise and counter-clockwise movements to produce images with bilateral symmetry that can be associated with faces (central nose or muzzle, eyes on either side of that, sometimes ears above that and sometimes a mouth below. Incidentally the bilateral property can be tested with this image, as faces can be again be easily seen when the image is rotated 180 degrees, but are much less apparent or very distorted at 90 degrees or other rotations. The previous figure also has some faces, but not as many as this figure.